Divisibility Rules 1 to 13

Divisibility rules or Divisibility test have been mentioned to make the division procedure easier and quicker. If students will learn the division rules in Maths or the divisibility tests for 1 to 20, they can solve the problems in a better way. For example, divisibility rules for 13 help us to know which numbers are completely divided by 13. Some of the numbers like 2, 3, 4, 5 have rules which can be understood easily. But rules for 7, 11, 13, are little complex and need to be understood elaborately.

Divisibility Test (Division Rules in Maths)

As the name suggests, divisibility tests or division rules in Maths help one to check whether a number is divisible by another number without actual division. If a number is completely divisible by another number it means that in such a case quotient will be a whole number and the division will leave zero as remainder.

Since every number is not completely divisible by every other number such numbers leave remainder other than zero. These rules are certain one which helps us to determine the actual divisor of a number just by considering the digits of the number. Let us look into these rules for different whole numbers one by one.

Divisibility Rules 1 to 13

The division rules from 1 to 13 in Maths are explained here in detail with many solved examples. Go through the below article to learn the shortcut methods to divide the numbers easily.

Divisibility by 1

Every number is divisible by 1. Divisibility rule for 1 doesn’t have any particular condition. Any number divided by 1 will give the number itself, irrespective of how large the number is. For example, 3 is divisible by 1 and 3000 is also divisible by 1 completely.

Divisibility by 2

Any even number or number whose last digit is an even number i.e.  2,4,6,8 including 0 is always completely divisible by 2.

Example: 508 is an even number and divisible by 2 but 509 is not an even number, hence not divisible by 2. Procedure to check whether 508 is divisible by 2 or not is as follows:

• Consider the number 508
• Just take the last digit 8 and divide it by 2
• If the last digit 8 is divisible by 2 then the number 508 is also divisible by 2.

Divisibility Rules for 3

Divisibility rule for 3 states that a number is completely divisible by 3 if the sum of its digits is divisible by 3 i.e., it is a multiple of 3

Consider a number, 308. To check whether 308 is divisible by 3 or not, take sum of the digits (i.e. 3+0+8= 11). Now check whether the sum is divisible by 3 or not. If the sum is a multiple of 3 then the original number is also divisible by 3. Here, since 11 is not divisible by 3, 308 is also not divisible by 3.

Similarly, 516 is divisible by 3 completely as the sum of its digits i.e. 5+1+6=12, is a multiple of 3.

Divisibility by 4

If the last two digits of a number are divisible by 4, then that number is a multiple of 4 and is divisible by 4 completely.

Example: Take the number 2308. Consider the last two digits i.e.  08. As 08 is divisible by 4, the original number 2308 is also divisible by 4.

Divisibility by 5

Numbers with last digit 0 or 5 are always divisible by 5.
Example: 10, 10000, 10000005, 595, 396524850 etc.

Divisibility by 6

Numbers which are divisible by both 2 and 3 are divisible by 6. That is, if last digit of the given number is even and the sum of its digits is a multiple of 3, then the given number is also a multiple of 6.

Example: 630, the number is divisible by 2 as the last digit is 0.
The sum of digits is 6+3+0 = 9, which is also divisible by 3.
Hence 630 is divisible by 6.

Divisibility Rules for 7

The rule for divisibility by 7 is given below:

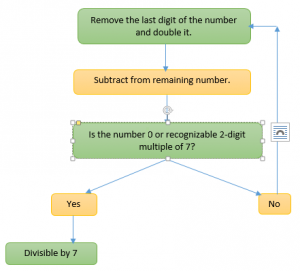
Example: Is 1073 divisible by 7?

• From the rule stated remove 3 from the number and double it, which becomes 6.
• Remaining number becomes 107, so 107-6 = 101.
• Repeating the process one more times, we have 1 x 2 = 2.
• Remaining number 10 – 2 = 8.
• As 8 is not divisible by 7, hence the number 1073 is not divisible by 7.

Divisibility by 8

If the last three digits of a number are divisible by 8, then the number is completely divisible by 8.

Example: Take number 24344. Consider the last two digits i.e.  344. As 344 is divisible by 8, the original number 24344 is also divisible by 8.

Divisibility by 9

The rule for divisibility by 9 is similar to divisibility rule for 3. That is, if the sum of digits of the number is divisible by 9, then the number itself is divisible by 9.

Example: Consider 78532, as the sum of its digits (7+8+5+3+2) is 25, which is not divisible by 9, hence 78532 is not divisible by 9

Divisibility by 10

Divisibility rule for 10 states that any number whose last digit is 0, is divisible by 10.

Example: 10, 20,30,1000,5000,60000 etc.

Divisibility Rules for 11

If the difference of the sum of alternative digits of a number is divisible by 11 then that number is divisible by 11 completely.

In order to check whether a number like 2143 is divisible by 11 following is the procedure.

• Group the alternative digits i.e. digits which are in odd places together and digits in even places together. Here 24 and 13 are two groups.
• Take the sum of the digits of each group i.e. 2+4=6 and 1+3= 4
• Now find the difference of the sums; 6-4=2
• If the difference is divisible by 11, then the original number is also divisible by 11. Here 2 is the difference which is not divisible by 11.
• Therefore, 2143 is not divisible by 11.

Divisibility Rules for 13

For any given number, to check if it is divisible by 13, we have to add four times of the last digit of the number to the remaining number and repeat the process until you get a two-digit number.  Now check if that two-digit number is divisible by 13 or not. If it is divisible then the given number is divisible by 13.

For example: 2795 → 279 + (5 x 4) → 279 + (20) → 299 → 29 + (9 x 4) → 29 + 36 →65. Number 65 is divisible by 13, 13 x 5 = 65.

Divisibility Rules Solved Examples

Example 1:

Check if 288 is divisible by 2.

Solution:

Given, 288 is a number.

If the last digit of 288 is divisible by 2, then 288 is also divisible by 2.

The last digit of 288 is 8, which is divisible by 2, such that;

8/2 = 4

Hence, 288 satisfy the divisibility rule for 2.

Example 2:

Check is 195 is divisible by 4 or not.

Solution:

As we can see, the last digit of 195 is 5, which is not divisible by 4.

Hence, 195 is not divisible by 4.